Uke 04: Laplace og Poissons likning
Læringsmål
Denne uken bruker vi Poissons og Laplace likning til å finne skalarpotensialet, \( V \), med forskjellige metoder.(AMS 4: Learning outcomes for this week: (1) Understand the use of Laplace and Poissons equations in one dimension, (2) Understand and use the uniqueness theorem, (3) Understand the use of Jacobi's method to solve Laplace equation.)
Diskusjonsoppgaver for gruppene
Exercise 5.1: Laplace operator
In one dimension, a linear curve is a solution to Laplace equation. Are there any other solutions that are not linear? In two dimensions, a plane may also be a solution to the Laplace equation. Are there any other solutions and what would that depend on?
(AMS 5: Provide a few curves. Talk about the Laplacian on these curves) . -->
Exercise 5.2: Laplace on a map
The image below is a surface showing a 3d rendering of Mount Everest (CC licence, Nate Loper) visualizing the height of the terrain as a function of position, \( h(x,y) \).
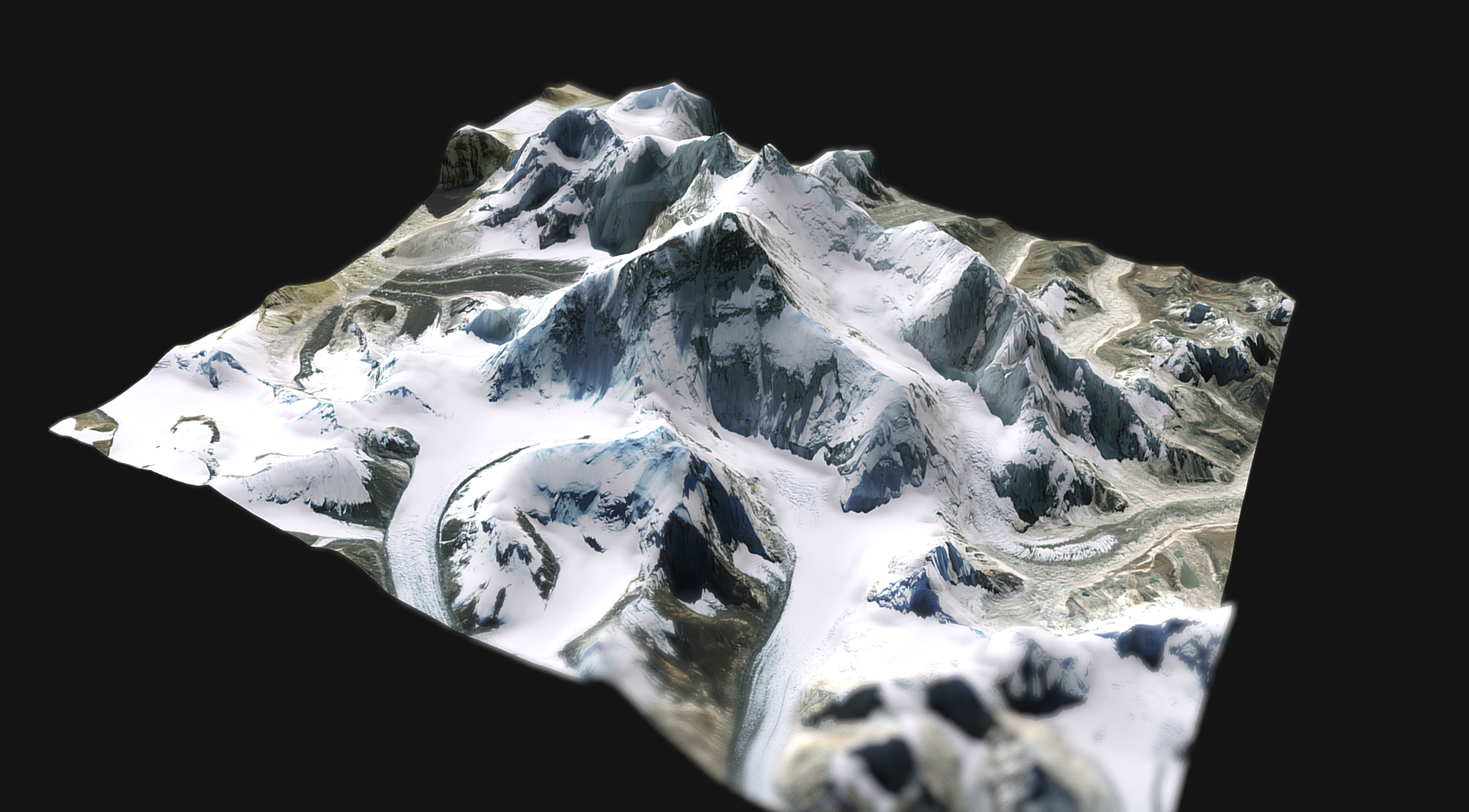
a) Discuss the sign of the Laplace operator, \( \nabla^2 h = \frac{\partial^2 h }{\partial x^2} + \frac{\partial^2 h}{\partial y^2} \), in various positions on the surface.
The image below is a surface showing a 3d rendering of a part of the Moon's surface (Image courtesy of NASA) visualizing the height of the terrain as a function of position, \( h(x,y) \).

b) Discuss the sign of the Laplace operator, \( \nabla^2 h = \frac{\partial^2 h }{\partial x^2} + \frac{\partial^2 h}{\partial y^2} \), in various positions on the surface.
c) In terms of the Laplace-operator how are the surfaces similar and different? (Can relate differences, to different geological processes affecting the two surfaces?)
Exercise 5.3: Discontinous potential
Is it possible to have a discontinuous potential? Provide examples or arguments for why it is not possible. (Hint: \( \vec{E} = - \nabla V \)).
Gruppeoppgaver
Exercise 5.4: Laplace operator on known potential surfaces
We know that the electric field from an infinite point charge \( Q \) in the origin is $$ \begin{equation} E_r = \frac{Q}{4 \pi \epsilon_0 r} \; , \tag{5.1} \end{equation} $$ We use this to visualize the potential from three possible configuration.
a) The first situation is illustrated in the figure below. What charge configuration does this correspond to? Check that it seems reasonable that \( \nabla^2 V = 0 \) in various points on the surface by examining the plot.
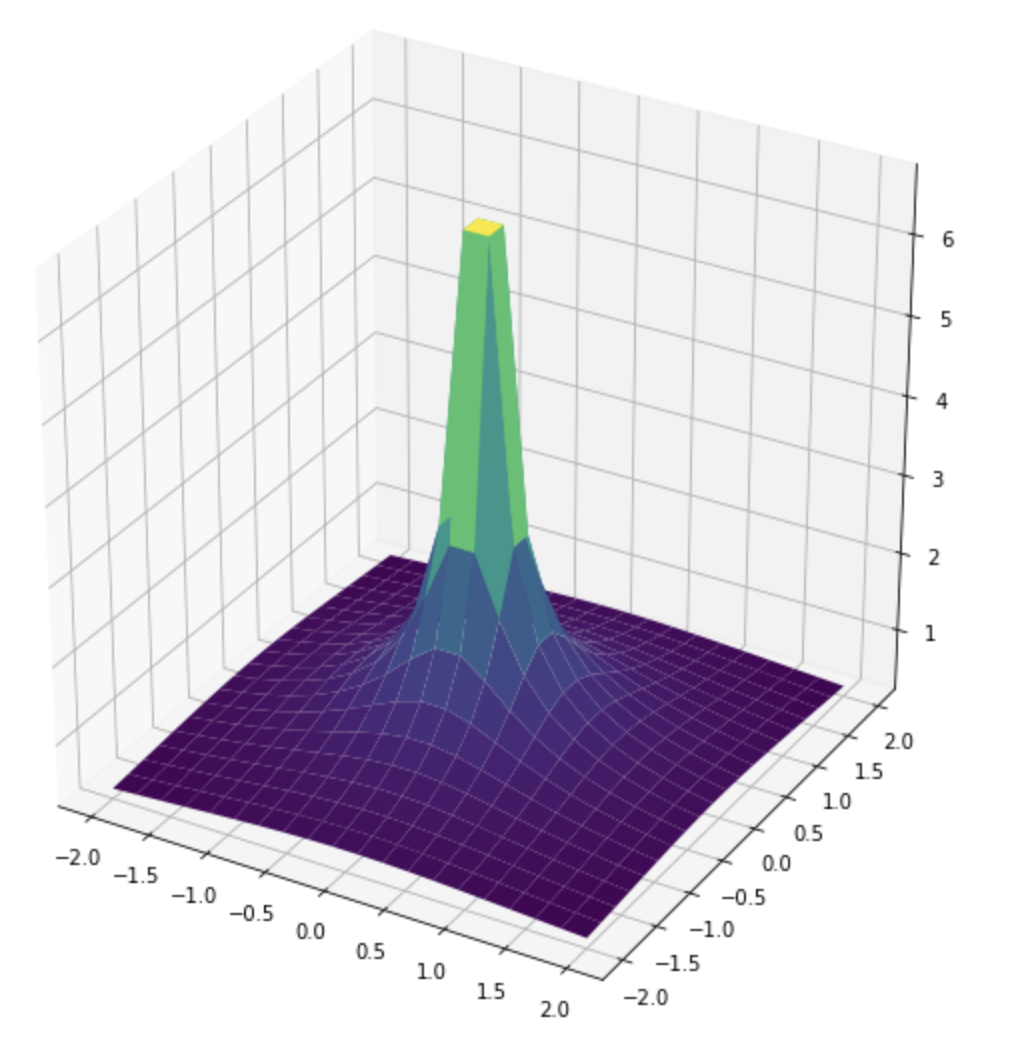
A positive charge at \( (0,0) \).
b) The first situation is illustrated in the figure below. What charge configuration does this correspond to? Check that it seems reasonable that \( \nabla^2 V = 0 \) in various points on the surface by examining the plot.
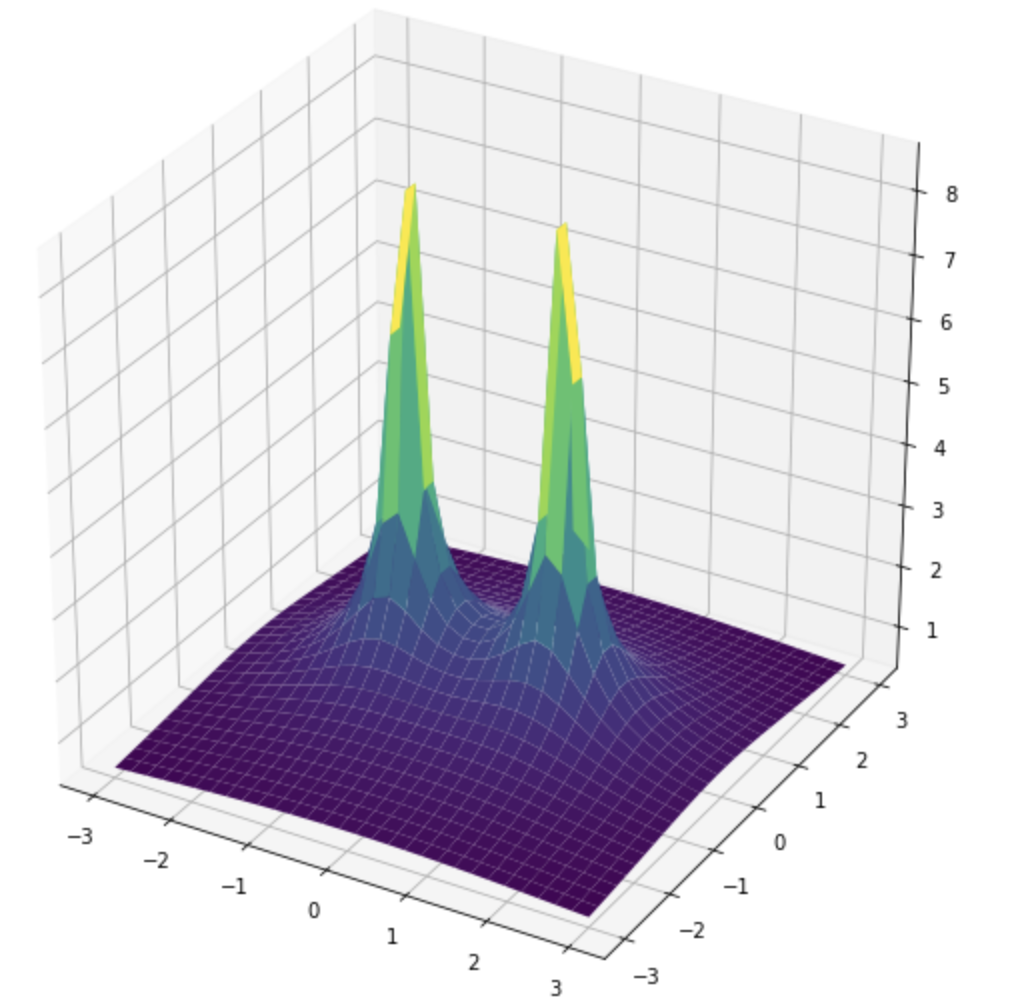
Two positive charges at \( (1,0) \) and \( (-1,0) \).
c) The first situation is illustrated in the figure below. What charge configuration does this correspond to? Check that it seems reasonable that \( \nabla^2 V = 0 \) in various points on the surface by examining the plot.
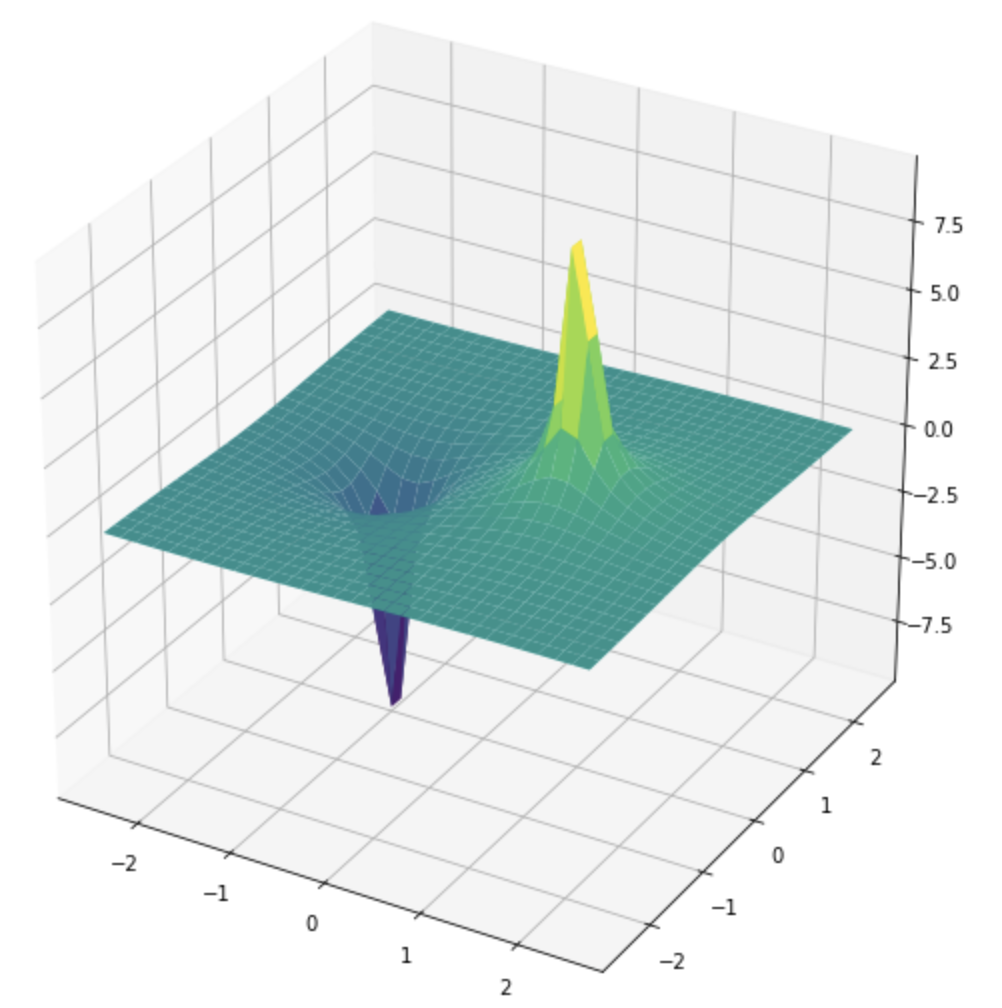
A positive charge at \( (1,0) \) and a negative charge of equal magnitude at \( (-1,0) \).
Exercise 5.5: Spatial variation of potentials (Laplace equation)
We will study a system in the \( xy \)-plane. At \( x=0 \) and \( x=L \) the scalar potential is \( V_0 = 0 \). The volume charge density between \( x=0 \) and \( x=L \) is zero, and the dielectric constant is \( \epsilon \).
a) Make a sketch of the system. What quantities is it useful to include in the sketch?
b) What is the scalar potential in the region between \( x=0 \) and \( x=L \)? Be very precise in how you determine this and what assumptions you make. What is the electric field in this region?
\( V(x,y) = 0 \)
There are no charges in this area. The electric scalar potential must satisfy Laplace equation \( \nabla^2 V = 0 \) with boundary conditions \( V(0,y) = 0 \) and \( V(L,y) = 0 \). The system is symmetric along the \( y \)-axis. We will therefore not have any \( y \)-dependence. Thus Laplace equation is \( \partial^2 V/\partial x^2 = 0 \). This has the solutions \( V(x) = Ax + B \). The boundary conditions gives that \( A = B = 0 \), and therefore \( V(x,y) = 0 \) for this system.
We now introduce a new feature in the system: The potential at \( x=L/2 \) is \( V_1 > V_0 \). The volume charge density is still zero, and the dielectric constant is \( \epsilon \).
c) Update your drawing to reflect this.
d) Find the scalar potential \( V(x,y) \) everywhere between \( x=0 \) and \( x=L \). What is the electric field?
\( V_1(x) = 2 V_1 x/L \) and \( E_1(x) = -2 V_1/L \) for \( 0 < x < L/2 \); \( V_2(x) = 2 (V_1/L) (L/2-x) + V_1 \) and \( E_2(x) = 2 V_1/L \) for \( L/2 < x < L \).
The system is still described by \( V(x,y) = A_ix + B_i \) in each of the regions (1) \( 0 < x < L/2 \) and (2) \( L/2 < x < L \). For the region \( 0 < x < L/2 \), the boundary conditions \( V(0) = 0 \) gives \( A_1 \cdot 0 + B_1 = 0 \) and therefore \( B_1 = 0 \). The other boundary condition \( V(L/2) = V_1 \) gives \( A_1(L/2) = V_1 \) and \( A_1 = 2 V_1 /L \), thus \( V(x) = 2 V_1 x/L \).
For the range \( L/2 < x < L \) we have that \( V(L) = 0 = A_2 L + B_2 \) and that \( V(L/2) = A_2 (L/2) + B_2 = V_1 \). Thus \( V(x) = 2 (V_1/L) (L/2-x) + V_1 \)
The electric field is \( E_1(x) = -\partial V/\partial x = -2 V_1/L \) for \( 0 < x < L/2 \) and \( E_2(x) = 2 V_1/L \) for \( L/2 < x < L \).
e) What kind of system could this situation represent?
A conductor with potential \( V_1 \) at \( x=L/2 \) and conductors at \( V=0 \) at \( x=0 \) and \( x=L \). (It can also be a sheet of charge at \( x=L/2 \). What would the charge be?)
f) What is the surface charge density on the surfaces at \( x=0 \), and \( x=L \). Discuss what happens at \( x=L/2 \).
\( \rho_a = 2 V_1 \epsilon/L \) on each side of \( x=L/2 \) and \( \rho_a = -2 V_1 \epsilon/L \) at \( x=0 \) and \( x=L \).
The surface charge density is given by the field, \( E_n = 2V_1/L = \rho_a/\epsilon \), and therefore \( \rho_a = 2 V_1 \epsilon/L \) at \( x=L/2 \) (on each side) and \( \rho_a = -2 V_1 \epsilon/L \) at \( x=0 \) and \( x=L \).
Exercise 5.6: Surface charges
Consider a sphere with radius \( a \) with a charge \( Q \) uniformly distributed on its surface.
a) What is the electric field as a function of \( r \)? (Find the behavior for both \( r < a \) and \( r > a \))
\( E=0 \) for \( r < a \) and \( \vec{E} = Q/(4 \pi \epsilon r^2) \hat{r} \) for \( r > a \).
We can use Gauss' law. We assume that the field will have spherical symmetry. Inside the sphere, there will be no charge inside a spherical surface, hence the flux and the field is zero. Outside the sphere, the flux is \( 4 \pi \epsilon R^2 E = Q \), and therefore \( E = Q/(4 \pi \epsilon R^2) \).
b) What is the scalar potential, \( V(r) \) ?
\( V(r) = Q/(4 \pi \epsilon r) \) for \( r > a \) and \( V(r) = Q/(4 \pi \epsilon a) \) for \( r \le a \).
The scalar potential is found from \( V(r) = \int_r^{\infty} E(r) \, dr = Q/(4 \pi \epsilon r) \) for \( r > a \). When \( r < a \) the field is zero, hence the potential is the same inside the sphere as on the sphere boundary, \( V=Q/(4 \pi\epsilon a) \).
c) Does \( V(r) \) satisfy Laplace equation? (Where does it/does it not satisfy the equation).
We find the \( \nabla^2 \) operator for spherical coordinates from the formula collection: $$ \begin{equation} \nabla^2V = \frac{1}{r^2} \frac{\partial}{\partial r} \left( r^2 \frac{\partial V}{\partial r} \right) + \frac{1}{r^2 \sin \theta}\frac{\partial}{\partial \theta} \left( \sin \theta \frac{\partial V}{\partial \theta } \right) + \frac{1}{r^2 \sin^2 \theta} \frac{\partial^V}{\partial \phi^2} \; . \tag{5.2} \end{equation} $$ Here, \( V \) has no \( \theta \) or \( \phi \) dependence. Hence, we insert the solution into \( \nabla^2 V \). We start from the inside: $$ \begin{equation} \frac{\partial V}{\partial r} = \frac{\partial}{\partial r} \frac{Q}{4 \pi \epsilon r} = -\frac{Q}{4 \pi r^2} \tag{5.3} \end{equation} $$ We see that if we multiply with \( r^2 \) we get a constant, and hence \( \nabla^2 V = 0 \).
For \( r < a \) the solution is constant and hence trivially satisfies Laplace equation.
d) Use your result for \( E \) to find the surface charge at \( r=a \).
\( \rho_a = Q/(4 \pi a^2) \)
We know that the change in field across \( r=a \) corresponds to the surface charge here: \( D_{2n} - D_{1n} = \rho_a \). Immediately inside the field is zero, and immediately outside it is \( D = E\epsilon = Q/(4 \pi \epsilon a^2)\epsilon = Q/(4 \pi a^2) \). Hence the surface charge density is \( \rho_a = Q/(4 \pi a^2) \), which is indeed what we could recognize immediately.
Exercise 5.7: Two-point boundary problems
In mechanics, we often solve initial value problems. For example, the equation of motion of a ball falling under gravity is a second-order ordinary differential equations, $$ \begin{equation*} \frac{d^2 x}{dt^2} = - g \quad \; . \end{equation*} $$ This equation has a unique solution given that you have two initial conditions, such as the position and velocity at a given time, \( x(t_0) = x_0 \) and \( v(t_0) = x'(t_0) = v_0 \).
In electromagnetism we also solve second-order differential equations. For example, if you want to find the electric potential in a region of space with a uniform charge distribution, \( \rho(x) = \rho_0 \), you know that the potential \( V(x) \) is the solution of Poisson's equation $$ \begin{equation*} \nabla^2 V = \frac{d^2V}{dx^2} = - \frac{\rho_0}{\epsilon_0} \; . \end{equation*} $$ This equation has a unique solution given that you have two boundary conditions, such as \( V(x_0) = V_0 \) and \( V(x_1) = V_1 \).
What is the difference between two initial condition and two boundary conditions? Let us address this for a specific example.
a) For a ball falling under gravity, assume that the initial position is \( x_0 = 0 \) and the initial velocity is \( v_0 = 10 \text{m/s} \). For simplicity, assume that \( g = 10 \text{m/s}^2 \). What is the position as a function of time?
\( x(t) = t \, 10 \text{m/s} - \frac{t^2}{2} 10 \text{m/s}^2 \)
The general solution is that \( x(t) = x_0 + v_0 t + \frac{1}{2}gt^2 \), which gives \( x(t) = t \, 10 \text{m/s} - \frac{t^2}{2} 10 \text{m/s}^2 \).
b) For the electromagnetic problem, let us assume that \( \rho_0/\epsilon_0 = -10 \text{V/m}^2 \) and that \( V(0) = 0 \) and that \( V(2) = 0 \). What is the electric potential as a function of position \( x \)? (All positions are measured in meters).
\( V(x) = x \, 10 \text{V/m}^2 - \frac{x^2}{2} 10 \text{V/m}^3 \)
The solution to Poisson's equation \( d^2/dx^2 = -\rho_0/\epsilon_0 \) must have the form $$ \begin{equation*} V(x) = Ax^2 + Bx + C \end{equation*} $$ where the coefficient \( A \) is determined by Poisson's equation, because \( \nabla^V = 2A = -10 \text{V/m}^3 \), and \( A = -\frac{1}{2}10 \text{V/m}^3 \). $$ \begin{equation*} V(x) = C + Bx -\frac{1}{2}10 x^2 \; . \end{equation*} $$ We determine \( B \) and \( C \) from the boundary conditions: \( V(0) = C = 0 \) and \( V(2) = 2B - 5\, 4 = 0 \), which gives \( B = 10\text{V/m}^2 \). The solution is therefore $$ \begin{equation*} V(x) = x \, 10 \text{V/m}^2 - \frac{x^2}{2} 10 \text{V/m}^3 \; . \end{equation*} $$
c) How does the solution change if you change the boundary conditions to be \( V(0) = 0 \) and \( V(2) = 2 \text{V/m} \)?
The boundary condition \( V(0)= 0 \) still gives that \( C=0 \). However, we now find that \( V(2) = 2B -20 = 2 \), which gives that \( B = 11 \text{V/m}^2 \). The solution is therefore $$ \begin{equation*} V(x) = x \, 11 \text{V/m}^2 - \frac{x^2}{2} 10 \text{V/m}^3 \; . \end{equation*} $$
d) How would you have to change the initial conditions for the mechanics problem to get a similar change in the solution?
\( v_0 = 11\text{m/s} \)
e) Can you choose any value for \( V(2) \) and still get a solution to Possion's equation?
Yes, \( C = 0 \) and \( B = (V(2)+20)/2 \text{V/m}^2 \).
f) You know that you can solve the initial value problem using Euler's or various Runge-Kutta methods. For example, Euler-Cromer's method works well: $$ \begin{align*} v(t + \Delta t) &\simeq v(t) + \Delta t \, a(t) \\ x(t + \Delta t) &\simeq x(t) + \Delta t +, v(t + \Delta t) \; . \end{align*} $$ Discuss what type of method you would have to use to solve the boundary value problem for Poisson's equation if you have a complex charge distribution \( \rho(x) \) so that you cannot find an analytical solution to the problem. (You are not expected to develop a numerical scheme, just discuss how you would try to develop such as scheme.)
The initial value problem is simple to solve numerically, since we simply step forward in time from the intial values. However, the boundary value problem is more tricky. We cannot start from \( x=0 \), because we do not know \( V'(0) \). Instead, we know where we should get after a given number of steps: \( V(0) = 0 \) and \( V(x_1) = V_1 \).
Hmmm. We need to approach this problem in a different way. One way is to discretize the equation $$ \begin{align*} \frac{d^2 V}{d x^2} &\simeq \frac{1}{\Delta x}\left( \frac{V(x+ \Delta x)-V(x)}{\Delta x} - \frac{V(x)-V(x-\Delta x)}{\Delta x} \right) \\ &\simeq \frac{V(x + \Delta x) + V(x-\Delta x) - 2 V(x)}{\Delta x^2} = - \rho(x)/\epsilon_0\; . \end{align*} $$ Let us introduce \( x_i = i \Delta x \) and \( V_i = V(x_i) \) so that \( V_0 \) is the boundary condition at \( x=0 \) and \( V_{N+1} = V_1 \) is the boundary condition at \( x_1 \). We can then list the equations as $$ \begin{align*} V_2+V_0-2V_1 &= -\Delta x^2 \, \rho(x_1)/\epsilon_0 \\ V_3+V_1-2V_2 &= -\Delta x^2 \, \rho(x_2)/\epsilon_0 \\ V_4+V_2-2V_3 &= -\Delta x^2 \, \rho(x_3)/\epsilon_0 \\ \ldots &= \ldots \\ V_{N}+V_{N-2}-2V_{N-1} &= -\Delta x^2 \, \rho(x_{N-1})/\epsilon_0 \\ V_{N+1}+V_{N-1}-2V_N &= -\Delta x^2 \, \rho(x_N)/\epsilon_0 \\ \end{align*} $$
This means that to solve this problem numerically, we need to solve this system of equations for \( V_i \). This is rather different than solving the problem with initial conditions.
Exercise 5.8: Laplace's equation in two dimensions
The Python programs provided in the text provides skeleton programs to solve Laplace's equation on a rectangular area with a given set of Dirichlet boundary conditions on the boudary \( S \), \( V(S) \).
a) Apply the program to the test case where \( V(x=0,y)=0 \) and \( V(x=L,y)=V_1 \). How can you check that the results are correct? Find the exact solution and compare with the numerical results.
b) Use the program to find \( V \) and \( \vec{E} \) for the case when \( V=0 \) for \( x=0 \) and \( x=L \), and \( V=V_1 \) for \( y=0 \) and \( y=L \). Discuss the potential and field you get. Does it correspond to your intuition?
c) Use the program to explore a situation of choice. Predict what you will get first, and then run the program to test your intuition.
Hjemmeoppgaver
Exercise 5.9: To metallplater
(HAS 6: Bearbeidet fra Johannes sin oppgave) Vi ser på et system som består av to plane metallplater med avstand \( d=1 \) cm. Platene er mye større enn avstanden mellom dem. Den ene platen er tilkoblet jord (\( V= 0 \)) mens den andre platen har et påtrykt positivt potensial \( V_0 \).
a) I første omgang er mediet mellom platene luft (\( \epsilon_r = 1 \)). Det oppstår gjennomslag i luften når feltstyrken overskrider \( E_{tl}=3\times 10^6 \) V/m. Hva er den største spenningen \( V_0 \) som kan påtrykkes mellom platene?
\( 3\cdot 10^4 \) V
For at det skal oppstå gjennomslag må spenningen være større enn \( V_{tl} = E_{tl} d = 3\cdot 10^4 \) V
b) Du tenger å påtrykke en større spenning enn dette. Derfor planlegger du å fylle luftlomma mellom platene med en \( 1.0 \) cm tykk plate av porselen, som har en relativ permittivitet på \( \epsilon_r=7 \) og tåler en feltstyrke på \( E_{tp}=6\cdot 10^6 \) V/m.
Hvor stor spenning beregner du at du nå kan påtrykke mellom platene?
\( 6\cdot 10^4 \) V
Dette dielektriske mediet vil gi større spenningstoleranse fordi det tåler en høyere spenning: \( V_{tp} = E_{tp}d = 6\cdot 10^4 \) V. Det er også verdt å merke seg at det å legge inn et dielektrikum vil føre til at ladningen på kondensatorplaten vil øke med en faktor \( \epsilon_r \).
c) Du går på butikken for å kjøpe porselensplate, men de har bare en plate som er \( 9 \) mm tykk. Kan denne platen hjelpe deg? Begrunn svaret med en beregning.
Dette endrer situasjonen dramatisk, siden vi nå får en grenseflate mellom porselen og luft. Vi vet at \( D \)-feltet ikke bryr seg om permittiviteten til materialet, så vi kommer til å ha et konstant \( D \)-felt mellom platene. Dermed får vi at \( E_p = \frac{E_l}{\epsilon-r} \). Vi setter så opp uttrykket for spenningsfallet mellom metallplatene: $$ \begin{equation} V = E_l(d-d_p) + E_p d_p = E_l \left[ d-d_p \left(1-\frac{1}{\epsilon_r}\right)\right] \tag{5.4} \end{equation} $$
Siden \( E_p = \frac{E_l}{\epsilon-r} \) kommer vi til å ha det sterkeste \( E \)-feltet i lufta, og siden lufta attpåtil tåler mindre felt enn porselenet er det gjennomslag i lufta vi trenger å bekymre oss for. Derfor er den høyeste spenningen som kan tåles $$ \begin{align} V_{t} &= E_{tl} \left[ d-d_p \left(1-\frac{1}{\epsilon_r}\right)\right] \tag{5.5}\\ &= 3\times 10^6 \text{ V/m} \cdot (0.01\text{ m} -0.009\text{ m} \cdot (1-1/7)) \tag{5.6}\\ &= 6857 \text{ V} \tag{5.7} \end{align} $$
Dette er mye mindre enn vi tålte med bare luft imellom, og det skyldes at det dielektriske materialet tar mindre av spenningsfallet mellom platene pga høyere permittivitet. Dermet tvinges luften til å ta det meste av spenningsfallet, og det over en mye mindre distanse enn tidligere, slik at feltet blir større.
Exercise 5.10: Ladningsfordeling mellom metallplater
(HAS 6: Bearbeidet fra Johannes sin oppgave)
To plane metallplater med avstand \( d=1 \) cm har en jevnt fordelt romladningstetthet \( \rho_v = -10^{-5}\text{ C/m}^3 \) mellom seg. Mediet mellom platene har dessuten relativ permittivitet \( \epsilon_r=1 \). Den ene platen er tilkoblet jord (\( V=0 \)) mens den andre platen har et positivt potensial \( V_0 = 10 \) V.
a) Finn potensialet mellom platene som funksjon av \( x \) når vi antar at platene har uendelig stor utstrekning.
Poissons ligning gir en 2. ordens differensiallikning i en variabel. Løs denne med grensebetingelsene \( V(0) = V_0 \) og \( V(d) = 0 \).
Poissons likning er \( \nabla^2 V = -\frac{\rho}{\epsilon} \). Siden platene er store antar vi at \( V \) kun kommer til å være en funksjon av \( x \), slik at poissons likning reduseres til $$ \begin{equation} \frac{\partial^2 V}{\partial x^2} = -\frac{\rho}{\epsilon} \tag{5.8} \end{equation} $$ der \( \rho \) og \( \epsilon \) er konstanter i denne oppgaven.
Vi integrerer likningen to ganger, og får at $$ \begin{equation} V(x) = -\frac{\rho x^2}{2\epsilon} + c_1 x + c2. \tag{5.9} \end{equation} $$
Grensebetingelsene gir oss $$ \begin{align} V(0) &= c_2 = V_0 \tag{5.10}\\ V(d) &= -\frac{\rho x^2}{2\epsilon} + c_1 d + V_0 \tag{5.11} \end{align} $$ altså $$ \begin{align} c_1 &= \frac{\rho d}{2\epsilon} - \frac{V_0}{d} \tag{5.12}\\ c_2 &= V_0 \tag{5.13} \end{align} $$ Vi setter dette inn i den generelle løsningen og får $$ \begin{equation} V(x) = -\frac{\rho x^2}{2\epsilon} + \left(\frac{\rho d}{2\epsilon} - \frac{V_0}{d}\right)x + V_0 \text{ for } x \in [0,d] \tag{5.14} \end{equation} $$
b) Finn det elektriske feltet som funksjon av \( x \).
I elektrostatikken er generelt \( E=-\nabla V \). Siden vi har et endimensjonal system reduseres dette til $$ \begin{equation} \mathbf{E} = -\frac{\d V}{\d x}\x = \left[ \frac{\rho x}{\epsilon} - \left( \frac{\rho d}{2\epsilon} - \frac{V_0}{d} \right)\right]\x \tag{5.15} \end{equation} $$
Om vi setter inn for verdiene oppgitt i oppgaven, i tillegg til \( \epsilon = \epsilon_0 \approx 8.854 \cdot 10^{-12} \) F/m siden luft har omtrent samme permittivitet som vakuum, får vi $$ \begin{equation} \mathbf{E} \approx (-1.13 x+6.65)\x \text{ V/mm} \tag{5.16} \end{equation} $$
c) For hvilken verdi av \( x \) har potensialet sitt minimum? Finn \( V_{\text{min}} \).
\( V_{\text{min}} = -9.57 \) V
Potensialet \( V \) har ekstremalverdier der \( \frac{\d V}{\d x} = 0 \), dvs. \( E(x) = 0 \), som gir $$ \begin{equation} \frac{\rho x}{\epsilon} - \left( \frac{\rho d}{2\epsilon} -\frac{V_0}{d} \right) = 0. \tag{5.17} \end{equation} $$ Når vi løser denne likningen for \( x \) får vi $$ \begin{equation} x_{ekstremal} = \frac{2}{2}-\frac{V_0\epsilon}{\rho d} \approx 5.89 \text{ mm} \tag{5.18} \end{equation} $$
Vi ser at \( E \)-feltet er positivt til venstre for dette punktet, og negativt til høyre for dette punktet. Siden \( E = -\frac{\d V}{\d x} \) kan vi dermed konkludere at \( x_{ekstremal} \) er et bunnpunkt for potensialet. Dersom det var et topppunkt måtte vi ha lett etter ekstremalpunkter på randen av området.
Vi evaluerer \( V(x) \) i \( x_{min} = 5.89 \) mm, og finner at $$ \begin{equation} V_{min} = V(x_{min}) = -9.57 \text{ V} \tag{5.19} \end{equation} $$
d) Skissér potensialet \( V(x) \), og \( x \)-komponenten av det elektriske feltet som funksjon av \( x \).
Figuren under viser potensialet og feltet.

Koden som genererer plottet:
import numpy as np
import matplotlib.pyplot as plt
def V(x, d=0.01, V0=10, Vd=0, epsilon=8.854e-12, rho=-1e-5):
return -rho*x**2/(2*epsilon) + (rho*d/(2*epsilon) - V0/d)*x + V0
def E(x, d=0.01, V0=10, Vd=0, epsilon=8.854e-12, rho=-1e-5):
return rho*x/epsilon - (rho*d/(2*epsilon) - V0/d)
x = np.linspace(0, 0.01, 100)
plt.figure(figsize=(6, 6))
plt.subplot(211)
plt.axhline(0, color="k")
plt.plot(x*100, V(x))
plt.plot([0, 0.589], [-9.57, -9.57], "k--", linewidth=1)
plt.plot([0.589,0.589], [-9.57,0], "k--", linewidth=1)
plt.text(0.589, -10, "$V_{min} = -9.57$ V", verticalalignment="top", horizontalalignment="center")
plt.text(0.589, 1, "$x_{min}=5.89$ mm",horizontalalignment="center")
plt.ylim([-13, 11])
plt.xlabel("x (cm)")
plt.ylabel("V (V)")
plt.subplot(212)
plt.axhline(0, color="k")
plt.plot(x*100, E(x)/1000)
plt.plot([0,1], [0,0], "k--")
plt.plot([0.589,0.589], [-0.2,0.2], "k-")
plt.text(0.589, 1, "$x_{min}$",horizontalalignment="center")
plt.xlabel("x (cm)")
plt.ylabel("E (V/mm)")
plt.tight_layout()
Exercise 5.11: Repetisjon: Feltet fra en ladet stav
(HAS 8: generisk oppgave) En ladet stav strekker seg fra \( -L \) til \( L \) på \( x \)-aksen med linjeladningstettehet \( \rho_l \).
a) Punktet \( P \) ligger på \( z \)-aksen med koordinatene \( (0,0,z) \). Finn det elektriske feltet fra staven i punktet \( P \).
Vi deler opp staven i små biter og bruker Coulombs lov på hver av de små bitene. $$ \begin{equation} \mathbf{E} = \lim_{\Delta x \to 0} \sum_i \frac{\rho_l \Delta x_i}{4\pi \epsilon_0 r_i^2} \frac{\rvec_i}{r_i} \tag{5.20} \end{equation} $$ Der summen er slik at den får med seg hele staven fra \( -L \) til \( L \).
Dette er nøyaktig definisjonen av følgende integral: $$ \begin{equation} \int_{-L}^L \frac{\rho_l \d x}{4\pi\epsilon_0 r^2}\frac{\rvec}{r} \tag{5.21} \end{equation} $$
der \( \rvec = -x\x + z\z \), altså vektoren som peker fra ladningen \( \rho_l \d x \) og mot punktet der vi ønsker å beregne feltet, og \( r = |\mathbf{r}| = \sqrt{x^2+z^2} \). Dermed blir integralet $$ \begin{equation} \mathbf{E} = \int_{x=-L}^L \frac{\rho_l \d x}{4\pi\epsilon_0 (x^2+z^2)^\frac{3}{2}}(-x\x + z\z) \tag{5.22} \end{equation} $$ Siden (-x) er antisymmetrisk på integrasjonsområdet \( [-L, L] \), og resten av uttrykket er symmetrisk på det samme området, kommer \( x \)-komponenten av feltet til å kansellere. Derfor ser vi bare videre på \( z \)-komponenten. $$ \begin{align} \mathbf{E} &= \int_{x=-L}^L \frac{z\rho_l \d x}{4\pi\epsilon_0 (x^2+z^2)^\frac{3}{2}}\z \tag{5.23}\\ &= \frac{\rho_l z\z}{4\pi\epsilon_0} \left[ \frac{x}{z^2 \sqrt{x^2+z^2}}\right]_{-L}^L \z \tag{5.24}\\ &= \frac{L\rho_l}{2\pi\epsilon_0 z \sqrt{z^2+L^2}}\z \tag{5.25}\\ &= \frac{Q}{4\pi\epsilon_0 z \sqrt{z^2+L^2}} \tag{5.26} \end{align} $$ Der vi i den siste likheten har skrevet \( Q = 2L\rho_l \) der \( Q \) er stavens totale ladning. Selve integralet løser vi enten ved å bruke sympy/wolframalpha, eller på egenhånd med integrasjonsteknikker. I dette integralet fører det fram å gjøre to substitusjoner etter hverandre: \( u = \frac{x}{z} \) og \( u = \tan\theta \). Da vil vi ende opp med \( \int \cos\theta \d \theta = \sin\theta + C \), og innsatt for hva \( \sin\theta \) er for noe, får vi løsningen.
b) Hvordan forventer du at feltet vil se ut langt fra staven, dvs. for \( |z| >> L \). Ser uttrykket du har for feltet fornuftig ut i denne grensen?
Når \( z \gg L \) vil \( z \) dominere over \( L \) inne i rottegnet slik at \( z\sqrt{z^2+L^2}\approx z^2 \). Dermed blir feltet for \( z \gg L \) $$ \begin{equation} \mathbf{E} \approx \frac{Q}{4\pi\epsilon_0 z^2}\z \tag{5.27} \end{equation} $$ som er det samme som feltet fra en punktladning. Dette gir mening, siden staven ser mer og mer ut som et punkt jo lenger unna man er.
c) Hvordan ser feltet ut for en uendelig lang stav (\( L\to \infty) \)? Når er dette uttrykket gyldig?
Vi tar grenseverdien $$ \begin{align} &\lim_{L\to\infty} \frac{L}{\sqrt{z^2+L^2}} \tag{5.28}\\ &= \lim_{L\to\infty} \frac{L}{L\sqrt{\frac{z^2}{L^2}+1}} \tag{5.29}\\ &= \lim_{L\to\infty} \frac{1}{\sqrt{\frac{z^2}{L^2}+1}} \tag{5.30}\\ &= 1 \tag{5.31} \end{align} $$ slik at feltet i grensen der \( L\to\infty \) blir $$ \begin{equation} \mathbf{E} = \frac{\rho_l}{2\pi\epsilon_0 z} \z \tag{5.32} \end{equation} $$ Da vi to grenseverdien så vi at det avgjørende var å få \( \frac{z^2}{L^2} \) til å bli liten, så dette uttrykket er gyldig dersom man er veldig nærme staven sammenlignet med stavens lengde. Altså \( z \ll L \).
Exercise 5.12: Modelling a 3D ion trap
(Based on an exercise by René Ask)
In 1989, Wolfgang Paul and Hans Georg Dehmelt received a Nobel prize in physics for the creation of the Paul trap (although the actual trapping technique was developed by them back in the 1950s). The trapping technique allows physicists to trap and study properties of particles and atoms. For example, at CERN physicists trap antiparticles using such a trap to measure their properties and compare them with theoretical predictions. Such traps can also be used to trap the fundamental computing units in quantum computers, known as qubits.
In this projecet, we will adress how to construct such a trap based from electric fields. Our goal is to trap ions with a charge \( q > 0 \) and mass \( m \). We'll assume that the trap is in a vacuum with no charge distibution within. The goal is to gradually develop a model for a trap, test the model numerically, explore the stability of the model, and realize the model in an experimental setup in the group sessions.
a) Imagine we want the particle to stay located in a small volume in space. The simplest such volume to represent mathematically would be a sphere, wouldn't it? To create a spherical trap, we can imagine that we set up a continuous distribution of charges to create a spherical harmonic oscillator (kind of like three decoupled springs with a mass attached to each) such that its electric potential is $$ \begin{equation} V(x,y,z) = A(x^2 + y^2 + z^2) \; , \tag{5.33} \end{equation} $$ where the constant \( A \) determines the strength of the potential. This way the particle would be limited to oscillate back and forth about the origin. Unfortunately, nature is not that simple. Show that the electric potential in (5.33) cannot exist in a vacuum.
Use Laplace's equation.
Laplace's equation is given by \( \nabla^2 V = 0 \) (recall that we assume there's no free charges inside the trap, so \( \rho = 0 \)). $$ \begin{equation} \nabla^2 V = \frac{\partial^2 V}{\partial x^2} + \frac{\partial^2 V}{\partial y^2} + \frac{\partial^2 V}{\partial z^2} = 6A \neq 0. \tag{5.34} \end{equation} $$ Since \( V \) does not obey Laplace's equation, it's an unphysical electric potential and cannot exist in a vacuum.
b) Now, let us see if we can modify the potential and create a potential that can exist in a vacuum, by modifying the form of the potential to be: $$ \begin{equation} V(x,y,z) = A(\alpha x^2 + \beta y^2 + \gamma z^2), \tag{5.35} \end{equation} $$ where \( \alpha, \beta, \gamma \neq 0 \) are constants that describe the potential. Let us find out how should we select these constants for the potential to be a physical possible potential in vacauum: Find a set of constraint on \( \alpha \), \( \beta \), and \( \gamma \) that ensures that the potential can exist in vacuum. (There is more than one choice). Prove that the potential $$ \begin{equation} V(x,y,z) = A( x^2 + y^2 - 2z^2) \; , \tag{5.36} \end{equation} $$ is a possible realization of these constraints and that it can exist in vacuum.
Again, use Laplace's equation.
\( \beta = \alpha \) and \( \gamma = -2\alpha \)
The potential must obey Laplace's equation, so $$ \begin{equation} \nabla^2 V =2A(\alpha + \beta + \gamma ) = 0, \tag{5.37} \end{equation} $$ which can be achieved if \( \beta = \alpha \) and \( \gamma = -2\alpha \) (there are several other valid constraints, but these will be more convenient with respect to the geometrical setup of the trap). Setting \( \alpha = 1 \), we get $$ \begin{equation} V(x,y,z) = A(x^2 + y^2 - 2z^2). \tag{5.38} \end{equation} $$
First attempt at constructing a trap
Figure 14 shows an experimental setup consisting of two finite paraboloid-shaped end-caps and a ring which will serve as electrodes. To trap positive ions, we want both end caps to be held at a constant positive potential \( V_0 \) and the ring to be held at a negative potential \( -V_0 \). The radius of the ring is \( r_0 \), and the tips of the end caps are placed at positions \( \pm z_0 \) relative to the center of the ring.
Figure 14: A simple setup for the trap with (approximately) the potential \( V(x,y,z) \). In reality the end caps must be paraboloids stretching out to infinity. Similarly, the ring must be a hyperboloid that stretches out to infinity in both directions.

c) We describe the potential using cylindrical coordinates \( x = r\cos\phi \), \( y = r\sin\phi \) and \( z = z \), with the origin in the center of the ring. In cylindrical coordinates the potential from these three electrodes approximately has the form: $$ \begin{equation} V(r,z) = A(r^2 - 2z^2) \; . \tag{5.39} \end{equation} $$ Find the constant \( A \) and the relationship between \( r_0 \) and \( z_0 \) so that the potential satisfies the boundary conditions on the ring and the tips of the end caps. Show that the potential for the trap can be written as: $$ \begin{equation} V(r,z) = \frac{V_0}{r_0^2}(2z^2 - r^2). \tag{5.40} \end{equation} $$ We will assume that this expression for \( V(r,z) \) is a good approximate model for the potential inside the between the electrods --- the region we will call the trap.
The ring is held at a negative potential \( -V_0 \), so \( V(r_0, 0) = -V_0 \) which implies $$ \begin{equation} V(r_0, 0) = Ar_0^2 = -V_0 \implies A = -\frac{V_0}{r_0^2} \; . \tag{5.41} \end{equation} $$ The end caps is held at a positive potential \( V_0 \), so \( V(0,z_0) = V_0 \), giving $$ \begin{equation} V(0, z_0) = \frac{V_0}{r_0^2}(2z_0^2) = V_0 \; , \tag{5.42} \end{equation} $$ meaning \( r_0^2 = 2z_0^2 \). Clearly, then, the potential can be written as $$ \begin{equation} V(r,z) = \frac{V_0}{r_0^2}(2z^2 - r^2) \; . \label{} \end{equation} $$
d) Provide an argument for why this potential also cannot trap a positive ion (Ah... that's a bummer, but worry not --- we will fix this later on).
You may do this in two ways: You can use a mathematical criterion in the form of the generalized second derivative test, the Hessian determinant, or you can plot the surface \( V(r,z) \) and use the surface plot to explain why you cannot trap the ion in this potential. You may need to reflect on how a potential needs to look in order to trap an ion.
The following Python program plots the potential \( V(r,z) \) as a surface or as a contour plot.
from mpl_toolkits.mplot3d import Axes3D
import matplotlib.pyplot as plt
import numpy as np
z0 = 1
r0 = np.sqrt(2)*z0
r = np.linspace(0, r0, 1001)
z = np.linspace(-z0, z0, 1001)
r,z = np.meshgrid(r,z)
V = 2*z*z - r*r
# Plot a surface
fig = plt.figure()
ax = fig.gca(projection="3d")
surf = ax.plot_surface(r,z,V)
plt.show()
# Plot a contour plot
plt.contourf(r,z,V)
plt.colorbar()
To interpret the contour plot, it may be useful to remember that the gradient \( \nabla V \) in cylindrical coordinates is $$ \begin{equation} \nabla V(r,\phi,z) = \frac{\partial V}{\partial r}\rhat + \frac{1}{r}\frac{\partial V}{\partial \phi} \phihat + \frac{\partial V}{\partial z}\z \; , \tag{5.43} \end{equation} $$ and that \( V(r,z) \) does not have any \( \phi \)-dependence.
The Hessian matrix is given by $$ \begin{equation} H = \begin{bmatrix} \frac{\partial^2 V}{\partial r^2} & \frac{\partial^2 V}{\partial r \partial z} \\ \frac{\partial^2 V}{\partial r \partial z} & \frac{\partial^2 V}{\partial z^2} \end{bmatrix} = \begin{bmatrix} -\frac{2V_0}{r_0^2} & 0 \\ 0 & \frac{4V_0}{r_0^2} \end{bmatrix} \tag{5.44} \end{equation} $$ which has the determinant \( \det H = -8V_0^2/r_0^4 < 0 \). Since \( \det H < 0 \), there exists no stable equilibrium for the potential and thus we can't possibly trap the ion.
The code below was used to produce the plot. In the figure, we can see that there's no stable equilibrium points since there are no local minima. But then we can't hope to successfully trap the particle since there's no point in space the particle will oscillate about.
from mpl_toolkits.mplot3d import Axes3D
import matplotlib.pyplot as plt
import numpy as np
fig = plt.figure()
ax = fig.gca(projection="3d")
z0 = 1
r0 = np.sqrt(2)*z0
r = np.linspace(0, r0, 1001)
z = np.linspace(-z0, z0, 1001)
r,z = np.meshgrid(r,z)
V = 2*z*z - r*r
surf = ax.plot_surface(r,z,V)
plt.show()
Demonstrating the instability of the trap
Let us demonstrate the instability of the trap by modeling the motion of an ion in the potential \( V(r,z) = (V_0/r_0^2)(2z^2 - r^2) \). First, we rewrite the potential in Cartesian coordinates by using that \( r^2 = x^2 + y^2 \), so that \( V(x,y,z) = (V_0/r_0^2)( 2z^2-x^2-y^2) \).
e) Show that the force, \( \vec{F} \), on an ion with charge \( q \) is $$ \begin{equation} \vec{F} = \frac{2 q V_0}{r_0^2} \left(x,y,-2z \right) \; . \tag{5.45} \end{equation} $$
We find the force from \( \vec{F} = q\vec{E} \), where \( \vec{E} = - \nabla V \). We see that : $$ \begin{equation} \frac{\partial V}{\partial x} = \frac{V^2}{r_0^2} (-x) \; , \; \frac{\partial V}{\partial y} = \frac{V^2}{r_0^2} (-y) \; , \; \frac{\partial V}{\partial z} = \frac{V^2}{r_0^2} (2z) \; , \tag{5.46} \end{equation} $$ which gives $$ \begin{equation} \vec{E} = - \nabla V = - \left(\frac{\partial V}{\partial x}, \frac{\partial V}{\partial y}, \frac{\partial V}{\partial z}\right) = \frac{V^2}{r_0^2}\left( x,y,-2z \right) \; , \tag{5.47} \end{equation} $$ and $$ \begin{equation} \vec{F} = q \vec{E} = \frac{qV^2}{r_0^2}\left( x,y,-2z \right) \; . \tag{5.48} \end{equation} $$
The following program finds the trajectory of an ion in this trap, with reasonable parameters for the trap: \( V_0 = 4000 \, \text{V} \), \( m = 5 \times 10^{-5} \, \text{kg} \) for a grain of cinnamon, \( q/m = 10^{-4} \, \text{C/kg} \), and \( z_0 = 0.005 \text{m} \).
import numpy as np
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import Axes3D
def particle_trajectory(Nsteps):
g = np.array([0,0,-9.81])
m = 5e-5 # mass of grain of cinnamon
V0 = 4000 # V
q_per_m = 1e-4 # C/kg
q = m*q_per_m
z0 = 0.005 # Typical size of trap
r0 = np.sqrt(2)*z0
dt = 1e-5 # Timestep
# Initialize trajectory
r = np.zeros((Nsteps,3))
v = np.zeros((Nsteps,3))
t = np.zeros(Nsteps)
r[0,:] = np.random.uniform(-0.5*z0,0.5*z0,size=3)
# Simulate motion
for i in range(Nsteps-1):
a = 2*q*V0/(m*r0**2)*np.array([r[i,0],r[i,1],-2*r[i,2]])+g
v[i+1,:] = v[i,:] + a*dt
r[i+1,:] = r[i,:] + v[i+1,:]*dt
t[i+1] = t[i] + dt
return r,v,t,z0
r,v,t,z0 = particle_trajectory(2000)
r = r/z0
fig = plt.figure()
ax = fig.gca(projection='3d')
ax.set_xlabel("x/z_0")
ax.set_xlabel("y/z_0")
ax.set_xlabel("z/z_0")
ax.plot(r[:,0], r[:,1], r[:,2],'k')
f) Plot a few trajectories for an ion. Plot the trajectories into a contour plot of \( V(r,z) \) and explain what you observe.
You can make a contour plot of \( V(r,z) \) with the following program:
import numpy as np
import matplotlib.pyplot as plt
z0 = 1
rs = np.linspace(0,4*z0,1001)
zs = np.linspace(-z0, z0, 1001)
rs,zs = np.meshgrid(rs,zs)
Vs = 2*zs*zs-rs*rs
plt.contourf(rs,zs,Vs)
plt.colorbar()
To interpret the contour plot, it may be useful to remember that the gradient \( \nabla V \) in cylindrical coordinates is $$ \begin{equation} \nabla V(r,\phi,z) = \frac{\partial V}{\partial r}\rhat + \frac{1}{r}\frac{\partial V}{\partial \phi} \phihat + \frac{\partial V}{\partial z}\z \; , \tag{5.49} \end{equation} $$ and that \( V(r,z) \) does not have any \( \phi \)-dependence.
Improving the trap
We may summarize our findings so far: a static electric field is not sufficient to trap charged particles in 3D. This is stated by Earnshaw's theorem. There's a clever way to fix this problem. Instead of working with a static potential, we replace the constant \( V_0 \) with the sinusoidally varying potential \( V_0 \cos (\Omega t) \). This way, if we provide an appropriate angular frequency \( \Omega \), we can stop the particle(s) in the trap from escaping in the \( xy \)-plane. The general expression is $$ \begin{equation} V(x,y,z,t) = \frac{V_0\cos \Omega t}{r_0^2}(2z^2 - x^2 - y^2) \; . \tag{5.50} \end{equation} $$
g) Show that the electric field corresponding to this potential is: $$ \begin{equation} \vec{E} = \frac{2V_0}{r_0^2}\cos\left( \Omega t \right) \left(x\x + y\y - 2z\z \right) \; . \tag{5.51} \end{equation} $$
We find the electric field from \( \vec{E} = - \nabla V \): $$ \begin{align} \vec{E} &=& -\nabla V \tag{5.52}\\ &=& \frac{2V_0}{r_0^2}\cos(\Omega t)(x\x + y\y - 2z\z ) \; . \tag{5.53} \end{align} $$
h) Find the force \( \vec{F} \) on a particle with charge \( q > 0 \) due to the electric field.
We find the force from \( \vec{F} = q \vec{E} \): $$ \begin {equation} \vec{F} = q\vec{E} = q\frac{2V_0}{r_0^2}\cos\left( \Omega t \right) \left(x\x + y\y - 2z\z \right) \; . \tag{5.54} \end{equation} $$
i) Rewrite the Python program to find the trajectory \( \vec{r}(t) \) of a particle and plot the trajectory in 3d for 10 trajectories. Can you trap the particle in this way?
For this problem you should use \( V_0 = 4000 \) V, \( \Omega = 100\pi \) Hz (that's the angular frequency a wall outlet would give you). In a demonstration of this type of trap one usually traps small, charged particles such as cinnamon. The mass of a cinnamon grain is roughly \( m \approx 5\times 10^{-5} \, \text{kg} \). The average charge per unit mass of a trapped cinnamon particle is roughly \( q/m = 10^{-4} \, \text{C/kg} \). A reasonable size for the trap is \( z_0 \approx 0.005 \ \text{m} \).
The following program find the trajectories:
import numpy as np
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import Axes3D
def particle_trajectory(Nsteps):
g = np.array([0,0,-9.81])
m = 5e-5 # mass of grain of cinnamon
V0 = 4000 # V
q_per_m = 1e-4 # C/kg
q = m*q_per_m
Omega = 2*np.pi*50 # Hz
dt = 1e-5 # Timestep
z0 = 0.005 # Typical size of trap
r0 = np.sqrt(2)*z0
# Initialize trajectory
r = np.zeros((Nsteps,3))
v = np.zeros((Nsteps,3))
t = np.zeros(Nsteps)
r[0,:] = np.random.uniform(-0.5*z0,0.5*z0,size=3)
v[0,:] = 10*np.random.uniform(-0.5*z0,0.5*z0,size=3)
# Simulate motion
for i in range(Nsteps-1):
a = 2*q*V0/(m*r0**2)*np.cos(Omega*t[i])*np.array([r[i,0],r[i,1],-2*r[i,2]])+g
v[i+1,:] = v[i,:] + a*dt
r[i+1,:] = r[i,:] + v[i+1,:]*dt
t[i+1] = t[i] + dt
return r,v,t,z0
# Plotting N trajectories
N = 10
fig = plt.figure()
ax = fig.gca(projection='3d')
for j in range(N):
r,v,t,z0 = particle_trajectory(20000)
r = r/z0
ax.plot(r[:,0], r[:,1], r[:,2])
The following figure shows 10 trajectories, which all are restricted to the region of the trap.

Challenge question (voluntary)
(This question is a bit more challenging, but is useful training if you are aiming for top grades at the exam.)
Test the stability of the trap by adding forces to reflect effects such as air flow from ventilation (wind), random kicks from brownian motion or interactions between the grain (more difficult). Choose one of these effects, or another effect you can argue for, implement the effect in the model, document your findings with plots, and discuss your findings briefly. Include your computer program in your answer.
Innleveringsoppgave (2 poeng)
Exercise 5.13: Laplace likning for en lang sylinder
I sylinderkoordinater er Laplace likning gitt som $$ \begin{equation} \nabla^2 V = \frac{1}{r}\frac{\partial}{\partial r}\left(r \frac{\partial V}{\partial r} \right) + \frac{1}{r^2} \frac{\partial^2 V}{\partial \phi^2} + \frac{\partial^2 V}{\partial z^2} = 0 \; . \tag{5.55} \end{equation} $$ Vi skal i denne oppgaven se på et uendelig langt sylinderskall som ligger langs \( z \)-aksen som en modell for en kabel eller aksonet i en nervecelle.
a) Forklar hvorfor potensialet \( V \) kun kan avhenge av \( r \) og ikke av \( \phi \) eller \( z \).
Dette systemet er sylindersymmetrisk. Systemet blir det samme om vi roterer det eller om vi forskyver det langs \( z \)-aksen. Derfor kan ikke potensialet endre seg langs \( z \)-aksen eller med \( \phi \). Potensialet er derfor \( V = V(r) \) og Laplace likning blir da $$ \begin{equation} \frac{1}{r}\frac{\partial}{\partial r}\left(r \frac{\partial V}{\partial r} \right) = 0 \; . \tag{5.56} \end{equation} $$
b) Vi antar at potensialet er \( V_0 \) på innsiden av sylinderskallet ved \( r = a \) og \( V_1=0 \) på utsiden av sylinderskallet ved \( r = b \). Det er ingen frie ladninger i området mellom \( r=a \) og \( r=b \). Finn det elektriske potensialet som funksjon av \( r \) ved å løse Laplace likning.
Laplace likning er i dette tilfellet: $$ \begin{equation} \frac{1}{r}\frac{\partial}{\partial r}\left(r \frac{\partial V}{\partial r} \right) = 0 \; . \tag{5.57} \end{equation} $$ For \( r>0 \) skriver vi det om til: $$ \begin{equation} \frac{\partial}{\partial r}\left(r \frac{\partial V}{\partial r} \right) = 0 \; . \tag{5.58} \end{equation} $$ Siden den deriverte med hensyn på \( r \) er null, er uttrykket inne i parantesen til venstre en konstant som vi kaller \( C_0 \): $$ \begin{equation} r \frac{\partial V}{\partial r} = C_0 \quad \Rightarrow \quad \frac{\partial V}{\partial r} = \frac{C_0}{r} \; . \tag{5.59} \end{equation} $$ Løsningen på denne likning kjenner vi igjen som logaritmen: $$ \begin{equation} V(r) = C_0 \ln r + C_1 \; , \tag{5.60} \end{equation} $$ hvor \( C_1 \) også er en konstant. Vi bestmmer \( C_0 \) og \( C_1 \) fra grensebetingelsene \( V(a) = V_0 \) og \( V(b) = 0 \). Vi begynner med \( V(b) = 0 \) som gir at \( C_0 \ln b + C_1 = 0 \) og dermed \( C_1 = - C_0 \ln b \) slik at \( V(r) = C_0 \ln r - C_0 \ln b = C_0 ( \ln r - \ln b) \). Vi setter dette inn i \( V(a) = V_0 = C_0 ( \ln a - \ln b) = C_0 \ln (a/b) \), slik at \( C_0 = V_0/\ln (a/b) \). Dermed blir løsningen $$ \begin{equation} V(r) = \frac{V_0}{\ln (a/b)} \left( \ln r - \ln b \right) \; . \tag{5.61} \end{equation} $$